Thermodynamics of Living Systems
It is widely
held that in the physical sciences the laws of thermodynamics have had a
unifying effect similar to that of the theory of evolution in the biological sciences.
What is intriguing is that the predictions of one seem to contradict the
predictions of the other. The second law of thermodynamics suggests a
progression from order to disorder, from complexity to simplicity, in the
physical universe. Yet biological evolution involves a hierarchical progression
to increasingly complex forms of living systems, seemingly in contradiction to
the second law of thermodynamics. Whether this discrepancy between the two
theories is only apparent or real is the question to be considered in the next
three chapters. The controversy which is evident in an article published in the
American Scientist 1 along with the replies it provoked
demonstrates the question is still a timely one.
The
First Law of Thermodynamics
Thermodynamics
is an exact science which deals with energy. Our world seethes with
transformations of matter and energy. Be these mechanical or chemical, the
first law of thermodynamics---the principle of the Conservation of
Energy---tells us that the total energy of the universe or any isolated part of
it will be the same after any such transformation as it was before. A major
part of the science of thermodynamics is accounting---giving an account of the
energy of a system that has undergone some sort of transformation. Thus, we
derive from the first law of thermodynamics that the change in the energy of a
system (
E) is equal to the work
done on (or by) the system (W) and the heat flow into
(or out of) the system (Q) Mechanical work and
energy are interchangeable, i.e., energy may be converted into mechanical work
as in a steam engine, or mechanical work can be converted into energy as in the
heating of a cannon which occurs as its barrel is bored. In mathematical terms
(where the terms are as previously defined):
E = Q + W 
(7-1)
The
Second Law of Thermodynamics
The second law
of thermodynamics describes the flow of energy in nature in processes which are
irreversible. The physical significance of the second law of thermodynamics is
that the energy flow in such processes is always toward a more uniform
distribution of the energy of the universe. Anyone who has had to pay utility
bills for long has become aware that too much of the warm air in his or her
home during winter escapes to the outside. This flow of energy from the house
to the cold outside in winter, or the flow of energy from the hot outdoors into
the air-conditioned home in the summer, is a process described by the second
law of thermodynamics. The burning of gasoline, converting energy
"rich" compounds (hydrocarbons) into energy "lean"
compounds, carbon dioxide (CO2) and water (H20), is a
second illustration of this principle.
The concept of entropy (S) gives us a more quantitative way to describe the
tendency for energy to flow in a particular direction. The entropy change for a
system is defined mathematically as the flow of energy divided by the
temperature, or,
S 
[Q / T] (7-2)
where S is the change in entropy,
Q is the heat flow into or
out of a system, and T is the absolute temperature in degrees Kelvin (K).
[Note: For a
reversible flow of energy such as occurs under equilibrium conditions, the
equality sign applies. For irreversible energy flow, the inequality applies.]
A Driving
Force
If we consider heat flow from a warm house to the outdoors on a cold winter
night, we may apply equation 7-2 as follows:
ST = Shouse + Soutdoors - Q / T1 + Q / T2 (7-3)
where Sr is the total
entropy change associated with this irreversible heat flow, T1 is
the temperature inside the house, and T2 is the temperature
outdoors. The negative sign of the first term notes loss of heat from the
house, while the positive sign on the second term recognizes heat gained by the
outdoors. Since it is warmer in the house than outdoors (T1 > T2),
the total entropy will increase (Sr > 0) as a
result of this heat flow. If we turn off the heater in the house, it will
gradually cool until the temperature approaches that of the outdoors, i.e., T1
= T2. When this occurs, the entropy change (S) associated with heat
flow (Q) goes to zero. Since
there is no further driving force for heat flow to the outdoors, it ceases;
equilibrium conditions have been established.
As this simple example shows, energy flow occurs in a direction that causes the
total energy to be more uniformly distributed. If we think about it, we can
also see that the entropy increase associated with such energy flow is
proportional to the driving force for such energy flow to occur. The second law
of thermodynamics says that the entropy of the universe (or any isolated system
therein) is increasing; i.e., the energy of the universe is becoming more
uniformly distributed.
It is often noted that the second law indicates that nature tends to go from
order to disorder, from complexity to simplicity. If the most random
arrangement of energy is a uniform distribution, then the present arrangement
of the energy in the universe is nonrandom, since some matter is very rich in
chemical energy, some in thermal energy, etc., and other matter is very poor in
these kinds of energy. In a similar way, the arrangements of mass in the
universe tend to go from order to disorder due to the random motion on an
atomic scale produced by thermal energy. The diffusional processes in the solid,
liquid, or gaseous states are examples of increasing entropy due to random
atomic movements. Thus, increasing entropy in a system corresponds to
increasingly random arrangements of mass and/or energy.
Entropy and Probability
There is another way to view entropy. The entropy of a system is a measure of
the probability of a given arrangement of mass and energy within it. A
statistical thermodynamic approach can be used to further quantify the system
entropy. High entropy corresponds to high probability. As a random arrangement
is highly probable, it would also be characterized by a large entropy. On the
other hand, a highly ordered arrangement, being less probable, would represent
a lower entropy configuration. The second law would tell us then that events
which increase the entropy of the system require a change from more order to
less order, or from less-random states to more-random states. We will find this
concept helpful in Chapter 9 when we analyze condensation reactions for DNA and
protein.
Clausius2, who formulated the second law of thermodynamics,
summarizes the laws of thermodynamics in his famous concise statement:
"The energy of the universe is constant; the entropy of the universe tends
toward a maximum." The universe moves from its less probable current
arrangement (low entropy) toward its most probable arrangement in which the
energy of the universe will be more uniformly distributed.
Life
and the Second Law of Thermodynamics
How does all of
this relate to chemical evolution? Since the important macromolecules of living
systems (DNA, protein, etc.) are more energy rich than their precursors (amino
acids, heterocyclic bases, phosphates, and sugars), classical thermodynamics
would predict that such macromolecules will not spontaneously form.
Roger Caillois has recently drawn this conclusion in saying, "Clausius and
Darwin cannot both be right."3 This prediction of classical
thermodynamics has, however, merely set the stage for refined efforts to
understand life's origin. Harold Morowitz4 and others have suggested
that the earth is not an isolated system, since it is open to energy flow from
the sun. Nevertheless, one cannot simply dismiss the problem of the origin of
organization and complexity in biological systems by a vague appeal to open-system
non-equilibrium thermodynamics. The mechanisms responsible for the emergence
and maintenance of coherent (organized) states must be defined. To clarify the
role of mass and energy flow through a system as a possible solution to
this problem, we will look in turn at the thermodynamics of (1) an isolated
system, (2) a closed system, and (3) an open system. We will then discuss the
application of open-system thermodynamics to living systems. In Chapter 8 we
will apply the thermodynamic concepts presented in this chapter to the
prebiotic synthesis of DNA and protein. In Chapter 9 this theoretical analysis
will be used to interpret the various prebiotic synthesis experiments for DNA
and protein, suggesting a physical basis for the uniform lack of success in synthesizing
these crucial components for living cells.
Isolated Systems
An isolated system is one in which neither mass nor energy flows in or out. To
illustrate such a system, think of a perfectly insulated thermos bottle (no heat
loss) filled initially with hot tea and ice cubes. The total energy in this
isolated system remains constant but the distribution of the energy changes
with time. The ice melts and the energy becomes more uniformly distributed in
the system. The initial distribution of energy into hot regions (the tea) and
cold regions (the ice) is an ordered, nonrandom arrangement of energy, one not
likely to be maintained for very long. By our previous definition then, we may
say that the entropy of the system is initially low but gradually increases
with time. Furthermore, the second law of thermodynamics says the entropy of
the system will continue to increase until it attains some maximum value, which
corresponds to the most probable state for the system, usually called
equilibrium.
In summary, isolated systems always maintain constant total energy while
tending toward maximum entropy, or disorder. In mathematical terms,
E / t = 0
(isolated system)
S / t 0 (7-4)
where E and S are the changes in the
system energy and system entropy respectively, for a time interval t. Clearly the emergence of
order of any kind in an isolated system is not possible. The second law of
thermodynamics says that an isolated system always moves in the direction of
maximum entropy and, therefore, disorder.
It should be noted that the process just described is irreversible in the sense
that once the ice is melted, it will not reform in the thermos. As a matter of
fact, natural decay and the general tendency toward greater disorder are so
universal that the second law of thermodynamics has been appropriately dubbed
"time's arrow."5
Closed Systems near Equilibrium
A closed system is one in which the exchange of energy with the outside world
is permitted but the exchange of mass is not. Along the boundary between the
closed system and the surroundings, the temperature may be different from the
system temperature, allowing energy flow into or out of the system as it moves
toward equilibrium. If the temperature along the boundary is variable (in
position but not time), then energy will flow through the system,
maintaining it some distance from equilibrium. We will discuss closed systems
near equilibrium first, followed by a discussion of closed systems removed from
equilibrium next.
If we combine the first and second laws as expressed in equations 7-1 and 7-2
and replace the mechanical work term W by P V, where P is pressure and V is volume change, we
obtain,
[NOTE: Volume
expansion (V> 0) corresponds to the
system doing work, and therefore losing energy. Volume contraction
(V 0) corresponds to work
being done on the system].
S [E + P V] / [T] (7-5)
Algebraic manipulation gives
E + P V - T S 
0 or G 0 (7-6)
where
G = E + P V - T S
The term on the left side of the
inequality in equation 7-6 is called the change in the Gibbs free energy (G). It may be thought of as
a thermodynamic potential which describes the tendency of a system to
change---e.g., the tendency for phase changes, heat conduction, etc. to occur.
If a reaction occurs spontaneously, it is because it brings a decrease in the
Gibbs free energy (G 0). This requirement is
equivalent to the requirement that the entropy of the universe increase. Thus,
like an increase in entropy, a decrease in Gibbs free energy simply means that
a system and its surroundings are changing in such a way that the energy of the
universe is becoming more uniformly distributed.
We may summarize then by noting that the second law of thermodynamics requires,
G / t 0, (closed system) (7-7)
where t indicates the time period
during which the Gibbs free energy changed.
The approach to equilibrium is characterized by,
G / t 
0, (closed system) (7-8)
The physical significance of equation 7-7
can be understood by rewriting equations 7-6 and 7-7 in the following form:
[S / t] - [ 1 / T (E / t + P V / t)] 0 (7-9)
or
(S / t ) - (1 / T H / t ) 0
and noting that the first term represents
the entropy change due to processes going on within the system and the second
term represents the entropy change due to exchange of mechanical and/or thermal
energy with the surroundings. This simply guarantees that the sum of the
entropy change in the system and the entropy change in the surroundings will be
greater than zero; i.e., the entropy of the universe must increase. For the
isolated system, E + P V = 0 and equation 7-9
reduces to equation 7-4.
A simple illustration of this principle is seen in phase changes such as water
transforming into ice. As ice forms, energy (80 calories/gm) is liberated to
the surrounding. The change in the entropy of the system as the amorphous water
becomes crystalline ice is -0.293 entropy units (eu)/degree Kelvin (K). The
entropy change is negative because the thermal and configuration entropy (or
disorder) of water is greater than that of ice, which is a highly ordered
crystal.
[NOTE:
Confirgurational entropy measures randomness in the distribution of matter in
much the same way that thermal entropy measures randomness in the distribution
of energy].
Thus, the
thermodynamic conditions under which water will transform to ice are seen from
equation 7-9 to be:
-0.293 - (-80 / T) > 0 (7-l0a)
or
T 273oK (7-l0b)
For condition of T 273oK
energy is removed from water to produce ice, and the aggregate disordering of
the surroundings is greater than the ordering of the water into ice crystals.
This gives a net increase in the entropy of the universe, as predicted by the
second law of thermodynamics.
It has often been argued by analogy to water crystallizing to ice that simple
monomers may polymerize into complex molecules such as protein and DNA. The
analogy is clearly inappropriate, however. The E + P V term (equation 7-9) in
the polymerization of important organic molecules is generally positive (5 to 8
kcal/mole), indicating the reaction can never spontaneously occur at or near
equilibrium.
[NOTE: If E + P V is positive, the entropy
term in eq 7 9 must be negative due to the negative sign which preceeds it. The
inequality can only be satisfied by S being sufficiently
positive, which implies disordenng].
By contrast the E + P V term in water changing to
ice is a negative, -1.44 kcal/mole, indicating the phase change is spontaneous
as long as T 273oK, as previously noted. The atomic bonding forces
draw water molecules into an orderly crystalline array when the thermal
agitation (or entropy driving force, T S) is made sufficiently
small by lowering the temperature. Organic monomers such as amino acids resist
combining at all at any temperature, however, much less in some orderly
arrangement.
Morowitz6 has estimated the increase in the chemical bonding energy
as one forms the bacterium Escherichia coli from simple precursors to be
0.0095 erg, or an average of 0.27 ev/ atom for the 2 x 1010 atoms in
a single bacterial cell. This would be thermodynamically equivalent to having
water in your bathtub spontaneously heat up to 360oC, happily a most
unlikely event. He goes on to estimate the probability of the spontaneous
formation of one such bacterium in the entire universe in five billion years under
equilibrium conditions to be 10-1011. Morowitz summarizes the
significance of this result by saying that "if equilibrium processes alone
were at work, the largest possible fluctuation in the history of the universe
is likely to have been no longer than a small peptide."7 Nobel
Laureate I. Prigogine et al., have noted with reference to the same
problem that:
The probability
that at ordinary temperatures a macroscopic number of molecules is assembled to
give rise to the highly ordered structures and to the coordinated functions
characterizing living organisms is vanishingly small. The idea of spontaneous
genesis of life in its present form is therefore highly improbable, even on the
scale of billions of years during which prebiotic evolution occurred.8
It seems safe to
conclude that systems near equilibrium (whether isolated or closed) can never
produce the degree of complexity intrinsic in living systems. Instead, they
will move spontaneously toward maximizing entropy, or randomness. Even the
postulate of long time periods does not solve the problem, as "time's
arrow" (the second law of thermodynamics) points in the wrong direction;
i.e., toward equilibrium. In this regard, H.F. Blum has observed:
The second law
of thermodynamics would have been a dominant directing factor in this case [of
chemical evolution]; the reactions involved tending always toward equilibrium,
that is, toward less free energy, and, in an inclusive sense, greater entropy.
From this point of view the lavish amount of time available should only have
provided opportunity for movement in the direction of equilibrium.9
(Emphasis added.)
Thus, reversing
"time's arrow" is what chemical evolution is all about, and this will
not occur in isolated or closed systems near equilibrium.
The possibilities are potentially more promising, however, if one considers a system
subjected to energy flow which may maintain it far from equilibrium, and its
associated disorder. Such a system is said to be a constrained system,
in contrast to a system at or near equilibrium which is unconstrained. The
possibilities for ordering in such a system will be considered next.
Closed Systems Far from Equilibrium
Energy flow through a system is the equivalent to doing work continuously on
the system to maintain it some distance from equilibrium. Nicolis and
Prigoginelo have suggested that the entropy change (S) in a system for a time
interval (t) may be divided into two
components.
S = Se + Si (7-11)
where Se is the entropy
flux due to energy flow through the system, and Si is the
entropy production inside the system due to irreversible processes such as
diffusion, heat conduction, heat production, and chemical reactions. We will
note when we discuss open systems in the next section that Se includes the
entropy flux due to mass flow through the system as well. The second law of
thermodynamics requires,
Si 0 (7-12)
In an isolated system, Se = 0 and
equations 7-11 and 7-12 give,
S =Si 0 (7-13)
Unlike Si, Se in a closed system
does not have a definite sign, but depends entirely on the boundary constraints
imposed on the system. The total entropy change in the system can be negative
(i.e., ordering within system) when,
Se 0 and | Se | > Si (7-14)
Under such conditions a state that would
normally be highly improbable under equilibrium conditions can be maintained
indefinitely. It would be highly unlikely (i.e., statistically just short of
impossible) for a disconnected water heater to produce hot water. Yet when the
gas is connected and the burner lit, the system is constrained by energy flow
and hot water is produced and maintained indefinitely as long as energy flows
through the system.
An open system offers an additional possibility for ordering---that of
maintaining a system far from equilibrium via mass flow through the system, as
will be discussed in the next section.
An open system is one which exchanges both energy and mass with the
surroundings. It is well illustrated by the familiar internal combustion
engine. Gasoline and oxygen are passed through the system, combusted, and then
released as carbon dioxide and water. The energy released by this mass flow
through the system is converted into useful work; namely, torque supplied to
the wheels of the automobile. A coupling mechanism is necessary, however, to
allow the released energy to be converted into a particular kind of work. In an
analagous way the dissipative (or disordering) processes within an open system
can be offset by a steady supply of energy to provide for (S) Se type work.
Equation 7-11, applied earlier to closed systems far from equilibrium, may also
be applied to open systems. In this case, the Se term
represents the negative entropy, or organizing work done on the system as a
result of both energy and mass flow through the system. This work done to the
system can move it far from equilibrium, maintaining it there as long as the
mass and/or energy flow are not interrupted. This is an essential
characteristic of living systems as will be seen in what follows.
Thermodynamics
of Living Systems
Living systems are
composed of complex molecular configurations whose total bonding energy is less
negative than that of their chemical precursors (e.g., Morowitz's estimate of E = 0.27 ev/atom) and whose
thermal and configurational entropies are also less than that of their chemical
precursors. Thus, the Gibbs free energy of living systems (see equation 7-6) is
quite high relative to the simple compounds from which they are formed. The formation
and maintenance of living systems at energy levels well removed from
equilibrium requires continuous work to be done on the system, even as
maintenance of hot water in a water heater requires that continuous work be
done on the system. Securing this continuous work requires energy and/or mass
flow through the system, apart from which the system will return to an
equilibrium condition (lowest Gibbs free energy, see equations 7-7 and 7-8)
with the decomposition of complex molecules into simple ones, just as the hot
water in our water heater returns to room temperature once the gas is shut off.
In living plants, the energy flow through the system is supplied principally by
solar radiation. In fact, leaves provide relatively large surface areas per
unit volume for most plants, allowing them to "capture" the necessary
solar energy to maintain themselves far from equilibrium. This solar energy is
converted into the necessary useful work (negative Se in equation
7-11) to maintain the plant in its complex, high-energy configuration by a
complicated process called photosynthesis. Mass, such as water and carbon
dioxide, also flows through plants, providing necessary raw materials, but not
energy. In collecting and storing useful energy, plants serve the entire
biological world.
For animals, energy flow through the system is provided by eating high energy
biomass, either plant or animal. The breaking down of this energy-rich biomass,
and the subsequent oxidation of part of it (e.g., carbohydrates), provides a
continuous source of energy as well as raw materials. If plants are deprived of
sunlight or animals of food, dissipation within the system will surely bring
death. Maintenance of the complex, high-energy condition associated with life
is not possible apart from a continuous source of energy. A source of energy
alone is not sufficient, however, to explain the origin or maintenance of
living systems. The additional crucial factor is a means of converting this
energy into the necessary useful work to build and maintain complex living
systems from the simple biomonomers that constitute their molecular building
blocks.
An automobile with an internal combustion engine, transmission, and drive chain
provides the necessary mechanism for converting the energy in gasoline into
comfortable transportation. Without such an "energy converter,"
however, obtaining transportation from gasoline would be impossible. In a
similar way, food would do little for a man whose stomach, intestines, liver,
or pancreas were removed. Without these, he would surely die even though he
continued to eat. Apart from a mechanism to couple the available energy to the
necessary work, high-energy biomass is insufficient to sustain a living system
far from equilibrium. In the case of living systems such a coupling mechanism
channels the energy along specific chemical pathways to accomplish a very
specific type of work. We therefore conclude that, given the availability of
energy and an appropriate coupling mechanism, the maintenance of a
living system far from equilibrium presents no thermodynamic problems.
In mathematical formalism, these concepts may be summarized as follows:
(1) The second law of thermodynamics requires only that the entropy production
due to irreversible processes within the system be greater than zero; i.e.,
Si > 0 (7-15)
(2) The maintenance of living systems
requires that the energy flow through the system be of sufficient magnitude
that the negative entropy production rate (i.e., useful work rate) that results
be greater than the rate of dissipation that results from irreversible
processes going on within the systems; i.e.,
| Se | > Si (7-16)
(3) The negative entropy generation must
be coupled into the system in such a way that the resultant work done is
directed toward restoration of the system from the disintegration that occurs
naturally and is described by the second law of thermodynamics; i.e.,
- Se = Si (7-17)
where Se and Si refer not
only to the magnitude of entropy change but also to the specific changes that
occur in the system associated with this change in entropy. The coupling must
produce not just any kind of ordering but the specific kind required by the
system.
While the maintenance of living systems is easily rationalized in terms of
thermodynamics, the origin of such living systems is quite another
matter. Though the earth is open to energy flow from the sun, the means of
converting this energy into the necessary work to build up living systems from
simple precursors remains at present unspecified (see equation 7-17). The
"evolution" from biomonomers of to fully functioning cells is the
issue. Can one make the incredible jump in energy and organization from raw
material and raw energy, apart from some means of directing the energy flow
through the system? In Chapters 8 and 9 we will consider this question, limiting
our discussion to two small but crucial steps in the proposed evolutionary
scheme namely, the formation of protein and DNA from their precursors.
It is widely agreed that both protein and DNA are essential for living systems
and indispensable components of every living cell today.11 Yet they
are only produced by living cells. Both types of molecules are much more energy
and information rich than the biomonomers from which they form. Can one
reasonably predict their occurrence given the necessary biomonomers and an
energy source? Has this been verified experimentally? These questions will be
considered in Chapters 8 and 9.
References
1. Victor F. Weisskopf, 1977. Amer. Sci. 65, 405-11.
2. R. Clausius, 1855. Ann. Phys. 125, 358.
3. R. Caillois, 1976. Coherences Aventureuses.
4. H.J. Morowitz, 1968. Energy Flow in Biology.
5. H.F. Blum, 1951. Time's Arrow and Evolution. Princeton:
6. H.J. Morowitz, Energy Flow, p.66.
7. H.J. Morowitz, Energy Flow, p.68.
8.
9. H.F. Blum, 1955. American Scientist 43, 595.
10. G. Nicolis and
11. S.L. Miller and L.E. Crgel, 1974. The Origins of Life on the Earth. Englewood
Cliffs,
Thermodynamics and the Origin of Life
Peter Molton has
defined life as "regions of order which use energy to maintain their
organization against the disruptive force of entropy."1 In
Chapter 7 it has been shown that energy and/or mass flow through a system can
constrain it far from equilibrium, resulting in an increase in order. Thus, it
is thermodynamically possible to develop complex living forms, assuming the
energy flow through the system can somehow be effective in organizing the
simple chemicals into the complex arrangements associated with life.
In existing living systems, the coupling of the energy flow to the organizing
"work" occurs through the metabolic motor of DNA, enzymes, etc. This
is analogous to an automobile converting the chemical energy in gasoline into
mechanical torque on the wheels. We can give a thermodynamic account of how
life's metabolic motor works. The origin of the metabolic motor (DNA, enzymes,
etc.) itself, however, is more difficult to explain thermodynamically, since a
mechanism of coupling the energy flow to the organizing work is unknown for
prebiological systems. Nicolis and Prigogine summarize the problem in this way:
Needless to say,
these simple remarks cannot suffice to solve the problem of biological order.
One would like not only to establish that the second law (dSi 0) is compatible with a
decrease in overall entropy (dS < 0), but also to indicate the mechanisms
responsible for the emergence and maintenance of coherent states.2
Without a doubt,
the atoms and molecules which comprise living cells individually obey the laws
of chemistry and physics, including the laws of thermodynamics. The enigma is
the origin of so unlikely an organization of these atoms and molecules. The
electronic computer provides a striking analogy to the living cell. Each
component in a computer obeys the laws of electronics and mechanics. The key to
the computer's marvel lies, however, in the highly unlikely organization of the
parts which harness the laws of electronics and mechanics. In the computer,
this organization was specially arranged by the designers and builders and
continues to operate (with occasional frustrating lapses) through the periodic
maintenance of service engineers.
Living systems have even greater organization. The problem then, that molecular
biologists and theoretical physicists are addressing, is how the organization
of living systems could have arisen spontaneously. Prigogine et al., have
noted:
All these
features bring the scientist a wealth of new problems. In the first place, one
has systems that have evolved spontaneously to extremely organized and complex
forms. Coherent behavior is really the characteristic feature of biological systems.3
In this chapter
we will consider only the problem of the origin of living systems.
Specifically, we will discuss the arduous task of using simple biomonomers to
construct complex polymers such as DNA and protein by means of thermal,
electrical, chemical, or solar energy. We will first specify the nature and
magnitude of the "work" to be done in building DNA and enzymes.
[NOTE: Work in
physics normally refers to force times displacement. In this chapter it refers
in a more general way to the change in Gibbs free energy of the system that
accompanies the polymerization of monomers into polymers].
In Chapter 9 we
will describe the various theoretical models which attempt to explain how the
undirected flow of energy through simple chemicals can accomplish the work
necessary to produce complex polymers. Then we will review the experimental
studies that have been conducted to test these models. Finally we will
summarize the current understanding of this subject.
How can we specify in a more precise way the work to be done by energy flow
through the system to synthesize DNA and protein from simple biomonomers? While
the origin of living systems involves more than the genesis of enzymes and DNA,
these components are essential to any system if replication is to occur. It is
generally agreed that natural selection can act only on systems capable of
replication. This being the case, the formation of a DNA/enzyme system by
processes other than natural selection is a necessary (though not sufficient)
part of a naturalistic explanation for the origin of life.
[NOTE: A
sufficient explanation for the origin of life would also require a model for
the formation of other critical cellular components, including membranes, and
their assembly].
Order
vs. Complexity in the Question of Information
Only recently
has it been appreciated that the distinguishing feature of living systems is
complexity rather than order.4 This distinction has come from the
observation that the essential ingredients for a replicating system---enzymes
and nucleic acids---are all information-bearing molecules. In contrast,
consider crystals. They are very orderly, spatially periodic arrangements of
atoms (or molecules) but they carry very little information. Nylon is another
example of an orderly, periodic polymer (a polyamide) which carries little
information. Nucleic acids and protein are aperiodic polymers, and this
aperiodicity is what makes them able to carry much more information. By
definition then, a periodic structure has order. An aperiodic structure has
complexity. In terms of information, periodic polymers (like nylon) and
crystals are analogous to a book in which the same sentence is repeated
throughout. The arrangement of "letters" in the book is highly
ordered, but the book contains little information since the information
presented---the single word or sentence---is highly redundant.
It should be noted that aperiodic polypeptides or polynucleotides do not necessarily
represent meaningful information or biologically useful functions. A random
arrangement of letters in a book is aperiodic but contains little if any useful
information since it is devoid of meaning.
[NOTE: H.P.
Yockey, personal communication, 9/29/82. Meaning is extraneous to the sequence,
arbitrary, and depends on some symbol convention. For example, the word
"gift," which in English means a present and in German poison,
in French is meaningless].
Only certain
sequences of letters correspond to sentences, and only certain sequences of
sentences correspond to paragraphs, etc. In the same way only certain sequences
of amino acids in polypeptides and bases along polynucleotide chains correspond
to useful biological functions. Thus, informational macro-molecules may be
described as being and in a specified sequence.5 Orgel notes:
Living organisms
are distinguished by their specified complexity.
Three sets of
letter arrangements show nicely the difference between order and complexity in
relation to information:
1. An ordered
(periodic) and therefore specified arrangement:
THE END THE END THE END THE END
Example: Nylon, or a crystal.
[NOTE: Here we
use "THE END" even though there is no reason to suspect that nylon or
a crystal would carry even this much information. Our point, of course, is that
even if they did, the bit of information would be drowned in a sea of
redundancy].
2. A complex (aperiodic) unspecified arrangement:
AGDCBFE GBCAFED ACEDFBG
Example: Random polymers (polypeptides).
3. A complex (aperiodic) specified arrangement:
THIS SEQUENCE OF LETTERS CONTAINS A
MESSAGE!
Example: DNA, protein.
Yockey7
and Wickens5 develop the same distinction, that "order" is
a statistical concept referring to regularity such as could might characterize
a series of digits in a number, or the ions of an inorganic crystal. On the
other hand, "organization" refers to physical systems and the
specific set of spatio-temporal and functional relationships among their parts.
Yockey and Wickens note that informational macromolecules have a low degree of
order but a high degree of specified complexity. In short, the redundant order
of crystals cannot give rise to specified complexity of the kind or magnitude
found in biological organization; attempts to relate the two have little
future.
Information
and Entropy
There is a
general relationship between information and entropy. This is fortunate because
it allows an analysis to be developed in the formalism of classical
thermodynamics, giving us a powerful tool for calculating the work to be done
by energy flow through the system to synthesize protein and DNA (if indeed
energy flow is capable of producing information). The information content in a
given sequence of units, be they digits in a number, letters in a sentence, or
amino acids in a polypeptide or protein, depends on the minimum number of
instructions needed to specify or describe the structure. Many instructions are
needed to specify a complex, information-bearing structure such as DNA.
Only a few instructions are needed to specify an ordered structure such
as a crystal. In this case we have a description of the initial sequence or
unit arrangement which is then repeated ad infinitum according to the
packing instructions.
Orgel9 illustrates the concept in the following way. To describe a
crystal, one would need only to specify the substance to be used and the way in
which the molecules were to be packed together. A couple of sentences would
suffice, followed by the instructions "and keep on doing the same,"
since the packing sequence in a crystal is regular. The description would be
about as brief as specifying a DNA-like polynucleotide with a random sequence.
Here one would need only to specify the proportions of the four nucleotides in
the final product, along with instructions to assemble them randomly. The
chemist could then make the polymer with the proper composition but with a
random sequence.
It would be quite impossible to produce a correspondingly simple set of
instructions that would enable a chemist to synthesize the DNA of an E. coli
bacterium. In this case the sequence matters. Only by specifying the
sequence letter-by-letter (about 4,000,000 instructions) could we tell a
chemist what to make. Our instructions would occupy not a few short sentences,
but a large book instead!
Brillouin,10 Schrodinger,11 and others12 have
developed both qualitative and quantitative relationships between information
and entropy. Brillouin,13 states that the entropy of a system is
given by
S = k ln 
(8-1)
where S is the entropy of the system, k
is Boltzmann's constant, and corresponds to the number
of ways the energy and mass in a system may be arranged.
We will use Sth and Sc to refer to the thermal and
configurational entropies, respectively. Thermal entropy, Sth, is
associated with the distribution of energy in the system. Configurational
entropy Sc is concerned only with the arrangement of mass in the
system, and, for our purposes, we shall be especially interested in the
sequencing of amino acids in polypeptides (or proteins) or of nucleotides in
polynucleotides (e.g., DNA). The symbols th and c refer to the
number of ways energy and mass, respectively, may be arranged in a system.
Thus we may be more precise by writing
S = k lnth c = k lnth + k lnc = Sth
+ Sc (8-2A)
where
Sth = k lnth (8-2b)
and
Sc = k lnc (8-2c)
Determining Information: From a Random
Polymer to an Informed Polymer
If we want to convert a random polymer into an informational molecule, we can
determine the increase in information (as defined by Brillouin) by finding the
difference between the negatives of the entropy states for the initial random
polymer and the informational molecule:
I = - (Scm - Scr) (8-3A),
I = Scr - Scm (8-3b),
= k lncr - k lncm (8-3c)
In this equation, I is a measure of the
information content of an aperiodic (complex) polymer with a specified
sequence, Scm represents the configurational "coding"
entropy of this polymer informed with a given message, and Scr
represents the configurational entropy of the same polymer for an unspecified
or random sequence.
[NOTE: Yockey
and Wickens define information slightly differently than Brilloum, whose
definition we use in our analysis. The difference is unimportant insofar as our
analysis here is concerned].
Note that the
information in a sequence-specified polymer is maximized when the mass in the
molecule could be arranged in many different ways, only one of which
communicates the intended message. (There is a large Scr from eq.
8-2c since cr is large, yet
Scm = 0 from eq. 8-2c since cm = 1.) The
information carried in a crystal is small because Sc is small (eq.
8-2c) for a crystal. There simply is very little potential for information in a
crystal because its matter can be distributed in so few ways. The random
polymer provides an even starker contrast. It bears no information
because Scr, although large, is equal to Scm (see eq.
8-3b).
In summary, equations 8-2c and 8-3c quantify the notion that only specified,
aperiodic macromolecules are capable of carrying the large amounts of
information characteristic of living systems. Later we will calculate "c" for both
random and specified polymers so that the configurational entropy change
required to go from a random to a specified polymer can be determined. In the
next section we will consider the various components of the total work required
in the formation of macromolecules such as DNA and protein.
DNA
and Protein Formation:
Defining
the Work
There are three distinct components of work to be done in assembling simple
biomonomers into a complex (or aperiodic) linear polymer with a specified
sequence as we find in DNA or protein. The change in the Gibbs free energy, G, of the system during
polymerization defines the total work that must be accomplished by energy flow
through the system. The change in Gibbs free energy has previously been shown
to be
G = E + P V - T S (8-4a)
or
G = H - T S (8-4b)
where a decrease in Gibbs free energy for
a given chemical reaction near equilibrium guarantees an increase in the
entropy of the universe as demanded by the second law of thermodynamics.
Now consider the components of the Gibbs free energy (eq. 8-4b) where the
change in enthalpy (H) is principally the
result of changes in the total bonding energy (E), with the (P V) term assumed to be
negligible. We will refer to this enthalpy component (H) as the chemical work.
A further distinction will be helpful. The change in the entropy (S) that accompanies the
polymerization reaction may be divided into two distinct components which
correspond to the changes in the thermal energy distribution (Sth) and the
mass distribution (Sc), eq. 8-2. So
we can rewrite eq. 8-4b as
G = H - TSth - T Sc (8-5)
that is,
(Gibbs free energy) = (Chemical work) - (Thermal entropy work) -
(Configurational entropy work)
It will be shown that polymerization of
macromolecules results in a decrease in the thermal and configurational
entropies (Sth 0, Sc 0). These terms
effectively increase G, and thus represent
additional components of work to be done beyond the chemical work.
Consider the case of the formation of protein or DNA from biomonomers in a
chemical soup. For computational purposes it may be thought of as requiring two
steps: (1) polymerization to form a chain molecule with an aperiodic but
near-random sequence, and (2) rearrangement to an aperiodic, specified
information-bearing sequence.
[NOTE: Some
intersymbol influence arising from differential atomic bonding properties makes
the distribution of matter not quite random. (H.P. Yockey, 1981. J. Theoret.
Biol. 91,13)].
The entropy
change (S) associated with the
first step is essentially all thermal entropy change (Sth), as
discussed above. The entropy change of the second step is essentially all
configurational entropy reducing change (Sc). In fact, as
previously noted, the change in configurational entropy (Sc) = Sc
"coding" as one goes from a random arrangement (Scr) to a
specified sequence (Scm) in a macromolecule is numerically equal to
the negative of the information content of the molecule as defined by Brillouin
(see eq. 8-3a).
In summary, the formation of complex biological polymers such as DNA and
protein involves changes in the chemical energy, H, the thermal entropy, Sth, and the
configurational entropy, Sc, of the
system. Determining the magnitudes of these individual changes using
experimental data and a few calculations will allow us to quantify the
magnitude of the required work potentially to be done by energy flow through
the system in synthesizing macromolecules such as DNA and protein.
Quantifying the Various Components of Work
1. Chemical Work
The polymerization of amino acids to polypeptides (protein) or of nucleotides to
polynucleotides (DNA) occurs through condensation reactions. One may calculate
the enthalpy change in the formation of a dipeptide from amino acids to be 5-8
kcal/mole for a variety of amino acids, using data compiled by Hutchens.14
Thus, chemical work must be done on the system to get polymerization to occur.
Morowitz15 has estimated more generally that the chemical work, or
average increase in enthalpy, for macromolecule formation in living systems is
16.4 cal/gm. Elsewhere in the same book he says that the average increase in
bonding energy in going from simple compounds to an E. coli bacterium is
0.27 ev/atom. One can easily see that chemical work must be done on the
biomonomers to bring about the formation of macromolecules like those that are
essential to living systems. By contrast, amino acid formation from simple
reducing atmosphere gases (methane, ammonia, water) has an associated enthalpy
change (H) of -50 kcal/mole to -250
kcal/ mole,16 which means energy is released rather than consumed.
This explains why amino acids form with relative ease in prebiotic simulation
experiments. On the other hand, forming amino acids from less-reducing
conditions (i.e., carbon dioxide, nitrogen, and water) is known to be far more
difficult experimentally. This is because the enthalpy change (H) is positive, meaning
energy is required to drive the energetically unfavorable chemical reaction
forward.
2.
Thermal Entropy Work
Wickens17
has noted that polymerization reactions will reduce the number of ways the
translational energy may be distributed, while generally increasing the
possibilities for vibrational and rotational energy. A net decrease results in
the number of ways the thermal energy may be distributed, giving a decrease in
the thermal entropy according to eq. 8-2b (i.e., Sth 0).
Quantifying the magnitude of this decrease in thermal entropy (Sth ) associated
with the formation of a polypeptide or a polynucleotide is best accomplished
using experimental results.
Morowitz18 has estimated that the average decrease in thermal
entropy that occurs during the formation of macromolecules of living systems in
0.218 cal/deg-gm or 65 cal/gm at 298oK. Recent work by Armstrong et
al.,19 for nucleotide oligomerization of up to a pentamer
indicates H and -T Sth values of
11.8 kcal/mole and 15.6 kcal/mole respectively, at 294K. Thus the decrease in
thermal entropy during the polymerization of the macromolecules of life
increases the Gibbs free energy and the work required to make these molecules,
i.e., -T Sth > 0.
3.
Configurational Entropy Work
Finally, we need
to quantify the configurational entropy change (Sc) that
accompanies the formation of DNA and protein. Here we will not get much help
from standard experiments in which the equilibrium constants are determined for
a polymerization reaction at various temperatures. Such experiments do not
consider whether a specific sequence is achieved in the resultant polymers, but
only the concentrations of randomly sequenced polymers (i.e., polypeptides)
formed. Consequently, they do not measure the configurational entropy (Sc) contribution
to the total entropy change (S). However, the magnitude
of the configurational entropy change associated with sequencing the polymers
can be calculated.
Using the definition for configurational "coding" entropy given in
eq. 8-2c, it is quite straightforward to calculate the configurational entropy
change for a given polymer. The number of ways the mass of the linear system
may be arranged (c) can be
calculated using statistics. Brillouin20 has shown that the number
of distinct sequences one can make using N different symbols and Fermi-Dirac
statistics is given by
= N! (8-6)
If some of these symbols are redundant
(or identical), then the number of unique or distinguishable sequences that can
be made is reduced to
c = N! / n1!n2!n2!...ni!
(8-7)
where n1 + n2 + ...
+ ni = N and i defines the number of distinct symbols. For a
protein, it is i =20, since a subset of twenty distinctive types of amino acids
is found in living things, while in DNA it is i = 4 for the subset of four
distinctive nucleotides. A typical protein would have 100 to 300 amino acids in
a specific sequence, or N = 100 to 300. For DNA of the bacterium E. coli, N
= 4,000,000. In Appendix 1, alternative approaches to calculating c are considered
and eq. 8-7 is shown to be a lower bound to the actual value.
For a random polypeptide of 100 amino acids, the configurational entropy, Scr,
may be calculated using eq. 8-2c and eq. 8-7 as follows:
Scr = k lncr
since cr = N! / n1!n2!...n20!
= 100! / 5!5!....5! = 100! / (5!)20
= 1.28 x 10115 (8-8)
The calculation of equation 8-8 assumes
that an equal number of each type of amino acid, namely 5, are contained in the
polypeptide. Since k, or Boltzmann's constant, equals 1.38 x 10-16
erg/deg, and ln [1.28 x 10115] = 265,
Scr = 1.38 x 10-16
x 265 = 3.66 x 10-14 erg/deg-polypeptide
If only one specific sequence of
amino acids could give the proper function, then the configurational entropy
for the protein or specified, aperiodic polypeptide would be given by
Scm = k lncm
= k ln 1
= 0
(8-9)
Determining scin Going from
a Random Polymer to an Informed Polymer
The change in configurational entropy, Sc, as one goes
from a random polypeptide of 100 amino acids with an equal number of each amino
acid type to a polypeptide with a specific message or sequence is:
Sc = Scm
- Scr
= 0 - 3.66 x 10-14 erg/deg-polypeptide
= -3.66 x 10-14 erg/deg-polypeptide (8-10)
The configurational entropy work (-T Sc) at ambient
temperatures is given by
-T Sc = - (298oK)
x (-3.66 x 10-14) erg/deg-polypeptide
= 1.1 x 10-11 erg/polypeptide
= 1.1 x 10-11 erg/polypeptide x [6.023 x 1023
molecules/mole] / [10,000 gms/mole] x [1 cal] / 4.184 x 107 ergs
= 15.8 cal/gm (8-11)
where the protein mass of 10,000 amu was
estimated by assuming an average amino acid weight of 100 amu after the removal
of the water molecule. Determination of the configurational entropy work for a
protein containing 300 amino acids equally divided among the twenty types gives
a similar result of 16.8 cal/gm.
In like manner the configurational entropy work for a DNA molecule such as for E.
coli bacterium may be calculated assuming 4 x 106 nucleotides in
the chain with 1 x 106 each of the four distinctive nucleotides,
each distinguished by the type of base attached, and each nucleotide assumed to
have an average mass of 339 amu. At 298oK:
-T Sc = -T (Scm
- Scr)
= T ( Scr - Scm)
= kT ln (cr - lncm)
= kT ln [(4 x 106)! / (106)!(106)!(106)!(106)!]
- kT ln 1
= 2.26 x 10-7 erg/polynucleotide
= 2.39 cal/gm 8-12
It is interesting to note that, while the
work to code the DNA molecule with 4 million nucleotides is much greater than
the work required to code a protein of 100 amino acids (2.26 x 10-7
erg/DNA vs. 1.10 x 10-11 erg/protein), the work per gram to code
such molecules is actually less in DNA. There are two reasons for this perhaps
unexpected result: first, the nucleotide is more massive than the amino acid
(339 amu vs. 100 amu); and second, the alphabet is more limited, with only four
useful nucleotide "letters" as compared to twenty useful amino acid
letters. Nevertheless, it is the total work that is important, which means that
synthesizing DNA is much more difficult than synthesizing protein.
It should be emphasized that these estimates of the magnitude of the
configurational entropy work required are conservatively small. As a practical
matter, our calculations have ignored the configurational entropy work involved
in the selection of monomers. Thus, we have assumed that only the proper subset
of 20 biologically significant amino acids was available in a prebiotic oceanic
soup to form a biofunctional protein. The same is true of DNA. We have assumed
that in the soup only the proper subset of 4 nucleotides was present and that
these nucleotides do not interact with amino acids or other soup ingredients.
As we discussed in Chapter 4, many varieties of amino acids and nucleotides
would have been present in a real ocean---varieties which have been ignored in
our calculations of configurational entropy work. In addition, the soup would
have contained many other kinds of molecules which could have reacted with
amino acids and nucleotides. The problem of using only the appropriate optical
isomer has also been ignored. A random chemical soup would have contained a
50-50 mixture of D- and L-amino acids, from which a true protein could
incorporate only the Lenantiomer. Similarly, DNA uses exclusively the optically
active sugar D-deoxyribose. Finally, we have ignored the problem of forming
unnatural links, assuming for the calculations that only CL-links occurred
between amino acids in making polypeptides, and that only correct linking at
the 3', 5'-position of sugar occurred in forming polynucleotides. A
quantification of these problems of specificity has recently been made by
Yockey.21
The dual problem of selecting the proper composition of matter and then
coding or rearranging it into the proper sequence is analogous to writing a story
using letters drawn from a pot containing many duplicates of each of the 22
Hebrew consonants and 24 Greek and 26 English letters all mixed together. To
write in English the message,
HOW DID I GET HERE?
we must first draw from the pot
In Chapter 6 we developed a scale showing degrees of investigator interference
in prebiotic simulation experiments. In discussing this scale it was noted that
very often in reported experiments the experimenter has actually played a
crucial but illegitimate role in the success of the experiment. It
becomes clear at this point that one illegitimate role of the investigator is
that of providing a portion of the configurational entropy work, i.e., the
"selecting" work portion of the total -T Sc work.
It is sometimes argued that the type of amino acid that is present in a protein
is critical only at certain positions---active sites---along the chain, but not
at every position. If this is so, it means the same message (i.e., function)
can be produced with more than one sequence of amino acids.
This would reduce the coding work by making the number of permissible
arrangements cm in eqs. 8-9
and 8-10 for Scm greater than 1. The effect of overlooking this in
our calculations, however, would be negligible compared to the effect of
overlooking the "selecting" work and only considering the
"coding" work, as previously discussed. So we are led to the
conclusion that our estimate for Sc is very
conservatively low.
Calculating the Total Work: Polymerization of Biomacromolecules
It is now possible to estimate the total work required to combine biomonomers
into the appropriate polymers essential to living systems. This calculation
using eq. 8-5 might be thought of as occurring in two steps. First, amino acids
polymerize into a polypeptide, with the chemical and thermal entropy work being
accomplished (H -T Sth). Next, the
random polymer is rearranged into a specific sequence which constitutes doing
configurational entropy work (-T Sc). For
example, the total work as expressed by the change in Gibbs free energy to make
a specified sequence is
G = H - T Sth - T Sc (8-13)
where H - T Sth may be
assumed to be 300 kcal/mole to form a random polypeptide of 101 amino acids
(100 links). The work to code this random polypeptide into a useful sequence so
that it may function as a protein involves the additional component of T Sc "coding"
work, which has been estimated previously to be 15.9 cal/gm, or approximately
159 kcal/mole for our protein of 100 links with an estimated mass of 10,000 amu
per mole. Thus, the total work (neglecting the "sorting and selecting"
work) is approximately
G = (300 + 159) kcal/mole =
459 kcal/mole (8-14)
with the coding work representing 159/459
or 35% of the total work.
In a similar way, the polymerization of 4 x 106 nucleotides into a
random polynucleotide would require approximately 27 x 106
kcal/mole. The coding of this random polynucleotide into the specified,
aperiodic sequence of a DNA molecule would require an additional 3.2 x 106
kcal/mole of work. Thus, the fraction of the total work that is required to
code the polymerized DNA is seen to be 8.5%, again neglecting the "sorting
and selecting" work.
The Impossibility of Protein Formation under Equilibrium Conditions
It was noted in Chapter 7 that because macromolecule formation (such as amino
acids polymerizing to form protein) goes uphill energetically, work must be
done on the system via energy flow through the system. We can readily see the
difficulty in getting polymerization reactions to occur under equilibrium
conditions, i.e., in the absence of such an energy flow.
Under equilibrium conditions the concentration of protein one would obtain from
a solution of 1 M concentration in each amino acid is given by:
K= [protein] x [H2 0] / [glycine]
[alanine]... (8-15)
where K is the equilibrium constant and
is calculated by
K = exp [ - G / RT ] (8-16)
An equivalent form is
G = -RT ln K (8-17)
We noted earlier that G = 459 kcal/mole for our
protein of 101 amino acids. The gas constant R = 1.9872 cal/deg-mole and T is
assumed to be 298oK. Substituting these values into eqs. 8-15 and
8-16 gives
protein concentration = 10-338
M (8-18)
This trivial yield emphasizes the
futility of protein formation under equilibrium conditions. In the next chapter
we will consider various theoretical models attempting to show how energy flow
through the system can be useful in doing the work quantified in this chapter
for the polymerization of DNA and protein. Finally, we will examine
experimental efforts to accomplish biomacromolecule synthesis.
References
1. Peter M.
Molton, 1978. J. Brit. Interplanet. Soc. 31, 147.
2. G. Nicolis and
3.
4. L.E. Orgel, 1973. The Origins of Life.
5. Yockey, J. Theoret. Biol, p.383.
6. Orgel, The Origins of Life, p.189.
7. Yockey, J. Theoret. Biol, p.579.
8. Wickens, J. Theoret. Biol., p.191.
9. Orgel, The Origins of Life, p.190.
10. L. Brillouin, 1951. J. Appi. Phys. 22, 334; 1951. J. Appl
Phys. 22, 338; 1950. Amer. Sci. 38, 5941949. Amer.
Sci. 37, 554.
11. E. Schrodinger, 1945. What is Life?
12. W. Ehrenberg, 1967. Sci. Amer. 217,108; Myron Tribus and Edward C.
McIrvine, 1971. Sci. Amer. 225, 197.
13. Brillouin, J. AppL Phys. 22, 885.
14. John 0. Hutchens, 1976. Handbook of Biochemistry and Molecular Biology, 3rd
ed., Physical and Chemical Data, Gerald D. Fasman.
15. H. Morowitz, 1968. Energy Flow in Biology.
16. H. Borsook and H.M. Huffman, 1944. Chemistry of Amino Acids and Proteins,
ed. C.L.A. Schmidt.
17. Wickens, J. Theoret. Biol, p.191.
18. Morowitz, Energy Flow in Biology, p.79.
19. D.W. Armstrong, F. Nome, J.H. Fendler, and J. Nagyvary, 1977. J. Mol.
Evol. 9, 218.
20. Brilllouin, J. AppL Phys. 22, 338.
21. H.P. Yockey, 1981. J. Theoret. Biol 91, 13.
Specifying How Work Is To Be Done
In Chapter 7 we saw that the work necessary to polymerize
DNA and protein molecules from simple biomonomers could potentially be
accomplished by energy flow through the system. Still, we know that such energy
flow is a necessary but not sufficient condition for polymerization of the
macromolecules of life. Arranging a pile of bricks into the configuration of a
house requires work. One would hardly expect to accomplish this work with
dynamite, however. Not only must energy flow through the system, it must be
coupled in some specific way to the work to be done. This being so, we devoted
Chapter 8 to identifying various components of work in typical polymerization
reactions. In reviewing those individual work components, one thing became
clear. The coupling of energy flow to the specific work requirements in the
formation of DNA and protein is particularly important since the required
configurational entropy work of coding is substantial.
Theoretical Models for the Origin of DNA and Protein
A mere appeal to open system thermodynamics does little
good. What must be done is to advance a workable theoretical model of how
the available energy can be coupled to do the required work. In this chapter
various theoretical models for the origin of DNA and protein will be evaluated.
Specifically, we will discuss how each proposes to couple the available energy
to the required work, particularly the configurational entropy work of coding.
Chance
Before the specified complexity of living systems began to be appreciated, it
was thought that, given enough time, "chance" would explain the
origin of living systems. In fact, most textbooks state that chance is the
basic explanation for the origin of life. For example, Lehninger in his classic
textbook Biochemistry
states,
We now come to the critical moment in evolution in which the
first semblance of "life" appeared, through the chance association of
a number of abiotically formed macromolecular components, to yield a unique
system of greatly enhanced survival value.1
More recently the viability of "chance" as a
mechanism for the origin of life has been severely challenged.2
We are now ready to analyze the "chance" origin of life using the
approach developed in the last chapter. This view usually assumes that energy
flow through the system is capable of doing the chemical and the thermal
entropy work, while the configurational entropy work of both selecting and
coding is the fortuitous product of chance.
To illustrate, assume that we are trying to synthesize a protein containing 101
amino acids. In eq. 8-14 we estimated that the total free energy increase (G) or work required to make
a random polypeptide from previously selected amino acids was 300 kcal/mole. An
additional 159 kcal/mole is needed to code the polypeptide into a protein.
Since the "chance" model assumes no coupling between energy flow and
sequencing, the fraction of the polypeptide that has the correct sequence may
be calculated (eq. 8-16) using equilibrium thermodynamics, i.e.,
[protein
concentration] / [polypeptide concentration] = exp ( - G / RT), eq. (9-1)
= exp (-159,000) / 1.9872 x 298)
or approximately 1 x 10-117
This ratio gives the fraction of polypeptides that have the right sequence
to be a protein.
[NOTE: This is essentially the inverse of the estimate for
the number of ways one can arrange 101 amino acids in a sequence (i.e., I / c in eq. 8-7)].
Eigen3 has estimated the number of polypeptides
of molecular weight 10 4 (the same weight used in our earlier
calculations) that would be found in a layer 1 meter thick covering the surface
of the entire earth. He found it to be 1041. If these polypeptides
reformed with new sequences at the maximum rate at which chemical reactions may
occur, namely 1014/s, for 5 x 109 years [1.6 x 1017
s], the total number of polypeptides that would be formed during the assumed
history of the earth would be
1041 x 1014/s
x 1.6 x 1017s = 1072 (9-2)
Combining the results of eq. 9-1 and 9-2, we find the probability of
producing one protein of 101 amino acids in five billion years is only 1/ 1045.
Using somewhat different illustrations, Steinman4 and Cairns-Smith5
also come to the conclusion that chance is insufficient.
It is apparent that "chance" should be abandoned as an acceptable
model for coding of the macromolecules essential in living systems. In fact, it
has been, except in introductory texts and popularizations.
Neo-Darwinian Natural Selection
The widespread recognition of the severe improbability that self-replicating
organisms could have formed from purely random interactions has led to a great
deal of speculation---speculation that some organizing principle must have been
involved. In the company of many others, Crick6 has considered that
the neo-Darwinian mechanism of natural selection might provide the answer. An
entity capable of self-replication is necessary, however, before natural
selection can operate. Only then could changes result via mutations and
environmental pressures which might in turn bring about the dominance of
entities with the greatest probabilities of survival and reproduction.
The weakest point in this explanation of life's origin is the great complexity
of the initial entity which must form, apparently by random fluctuations,
before natural selection can take over. In essence this theory postulates the
chance formation of the "metabolic motor" which will subsequently be
capable of channeling energy flow through the system. Thus harnessed by
coupling through the metabolic motor, the energy flow is imagined to supply not
only chemical and thermal entropy work, but also the configurational entropy
work of selecting the appropriate chemicals and then coding the resultant
polymer into an aperiodic, specified, biofunctioning polymer. As a minimum,
this system must carry in its structure the information for its own synthesis,
and control the machinery which will fabricate any desired copy. It is widely
agreed that such a system requires both protein and nucleic acid.7
This view is not unanimous, however. A few have suggested that a short peptide
would be sufficient.8
One way out of the problem would be to extend the concept of natural selection
to the pre-living world of molecules. A number of authors have entertained this
possibility, although no reasonable explanation has made the suggestion
plausible. Natural selection is a recognized principle of differential
reproduction which presupposes the existence of at least two distinct types of
self-replicating molecules. Dobzhansky appealed to those doing origin-of-life
research not to tamper with the definition of natural selection when he said:
I would like to plead with you, simply, please realize you
cannot use the words "natural selection" loosely. Prebiological
natural selection is a contradiction in terms.9
Bertalanffy made the point even more cogently:
Selection, i.e., favored survival of "better"
precursors of life, already presupposes self-maintaining, complex, open systems
which may compete; therefore selection cannot account for the origin of such
systems.10
Inherent Self-Ordering
Tendencies in Matter
How could energy flow through the system be sufficiently coupled to do the
chemical and thermal entropy work to form a nontrivial yield of polypeptides
(as previously assumed in the "chance" model)? One answer has been
the suggestion that configurational entropy work, especially the coding work,
could occur as a consequence of the self-ordering tendencies in matter. The
experimental work of Steinman and Cole11 in the late Sixties is
still widely cited in support of this model.12 The polymerization of
protein is hypothesized to be a nonrandom process, the coding of the
protein resulting from differences in the chemical bonding forces. For example,
if amino acids A and B react chemically with one another more readily than with
amino acids C, D, and E, we should expect to see a greater frequency of AB
peptide bonds in protein than AC, AD, AE, or BC, BD, BE bonds.
Together with our colleague Randall Kok, we have recently analyzed the ten
proteins originally analyzed by Steinman and Cole,13 as well as
fifteen additional proteins whose structures (except for hemoglobin) have been
determined since their work was first published in 1967. Our expectation in
this study was that one would only get agreement between the dipeptide bond
frequencies from Steinman and Cole's work and those observed in actual proteins
if one considered a large number of proteins averaged together. The distinctive
structures of individual proteins would cause them to vary greatly from
Steinman and Cole's data, so only when these distinctives are averaged out
could one expect to approach Steinman and Cole's dipeptide bond frequency
results. The reduced data presented in table 9-1 shows that Steinman and Cole's
dipeptide bond frequencies do not correlate well with the observed peptide bond
frequencies for one, ten, or twenty-five proteins. It is a simple matter to
make such calculations on an electronic digital computer. We surmise that
additional assumptions not stated in their paper were used to achieve the
better agreements.
Furthermore, the peptide bond frequencies for the twenty-five proteins approach
a distribution predicted by random statistics rather than the dipeptide bond
frequency measured by Steinman and Cole. This observation means that bonding
preferences between various amino acids play no significant role in coding
protein. Finally, if chemical bonding forces were influential in amino acid
sequencing, one would expect to get a single sequence (as in ice crystals) or
no more than a few sequences, instead of the large variety we observe in living
systems. Yockey, with a different analysis, comes to essentially the same
conclusion.14
A similar conclusion may be drawn for DNA synthesis. No one to date has
published data indicating that bonding preferences could have had any role in
coding the DNA molecules. Chemical bonding forces apparently have minimal
effect on the sequence of nucleotides in a polynucleotide.
Table 9-1.
Comparison of Steinman and Cole's experimentally determined
dipeptide bond frequencies, and frequencies calculated by Steinman and Cole,
and by Kok and Bradley from known protein sequences.
|
|
|
|
|
|
|||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
(Adapted after G. Steinman and M.V. Cole, 1967.
Proc. Nat. Acad. Sci. U.S. 58,735).
* The dipeptides are listed in terms of increasing volume of the side chains of
the constituent residues. Gly = glycine, Ala = alanine, Val = valine, Leu =
leucine, Ile = isoleucine and Phe = phenylalanine. Example: Gly-Ala =
glycylalanine.
+ Steinman and Cole's (S/C) experimentally determined dipeptide bond
frequencies were normalized and compared to the calculated frequencies obtained
by counting actual peptide bond frequencies in ten proteins, assuming all seryl
and threonyl residues are counted as glycine and all aspartyl and glutamyl
residues are counted as alanine. The ten proteins used were: egg lysozyme,
ribonuclease, sheep insulin, whale myoglobin, yeast cytochrome c, tobacco
mosaic virus, beta-corticotropin, glucagon, melanocyte-stimulating hormone, and
chymotrypsinogen. Because of ambiguity regarding sequences used by S/C, all
sequences are those shown in Atlas of Protein Sequence and Structure,
1972. Vol. V (ed. by M.O. Dayhoff). National Biomedical Research Foundation,
Georgetown University Medical Center, Washington, D.C.
& The experimentally determined dipeptide frequencies were obtained with
aqueous solutions containing 0.01 M each amino acid, 0.125 N HCl, 0.1 M sodium
dicyanamide.
#Kok and Bradley's (K/Bcalculated dipeptide frequencies were obtained by
counting S?Cassumptions. The numbers in brackets are for one protein,
enterotoxin B, with actual peptide bond frequencies for the same ten proteins
with (wa) and without (woa) S/C assumptions. The numbers in parentheses are for
twenty-five proteins with (wa) and without (woa) S/C assumptions. The
twenty-five proteins are the ten used S/C and alpha S1 Casein (bovine); azurin
(bordetella bronchisetica); carboxypeptidase A (bovine); cytochrome b5
(bovine); enterotoxin B; elastase (pig); glyceraldehyde 3-phosphate
dehydrogenase (lobster); human growth hormone; human hemoglobin beta chain;
histone 11B2 (bovine); immunoglobulin gamma-chain 1, V-I (human EU); penicillinase
(bacillus licheniformis 749/c); sheep prolactin; subtilisin (bacillus
amyloliquefaciens); and tryptophan synthetase alpha chain (E-coh
K-i 2). Sequences are those shown in Atlas of Protein Sequence and
Structure, 1972. Vol. V (ed. by M.O. Dayhoff). Note disagreement S/C K/B
calculated results. Also S/C calculated results are at variance with S/C
experimental values for one, ten or twenty-five proteins, with (wa) or without
(woa) S/C assumptions.
Mineral Catalysis
Mineral catalysis is often suggested as being significant in prebiotic
evolution. In the experimental investigations reported in the early 1970's15
mineral catalysis in polymerization reactions was found to operate by
adsorption of biomonomers on the surface or between layers of clay. Monomers
were effectively concentrated and protected from rehydration so that
condensation polymerization could occur. There does not appear to be any
additional effect. In considering this catalytic effect of clay, Hulett has
advised, "It must be remembered that the surface cannot change the free
energy relationships between reactants and products, but only the speed with
which equilibrium is reached."16
Is mineral catalysis capable of doing the chemical work and/or thermal entropy
work? The answer is a qualified no. While it should assist in doing the thermal
entropy work, it is incapable of doing the chemical work since clays do not
supply energy. This is why successful mineral catalysis experiments invariably
use energy-rich precursors such as aminoacyl adenylates rather than amino
acids.17
Is there a real prospect that mineral catalysis may somehow accomplish the
configurational entropy work, particularly the coding of polypeptides or
polynucleotides? Here the answer is clearly no. In all experimental work to
date, only random polymers have been condensed from solutions of selected
ingredients. Furthermore, there is no theoretical basis for the notion that
mineral catalysis could impart any significant degree of information content to
polypeptides or polynucleotides. As has been noted by Wilder-Smith,18
there is really no reason to expect the low-grade order resident on minerals to
impart any high degree of coding to polymers that condense while adsorbed on
the mineral's surface. To put it another way, one cannot get a complex,
aperiodic-sequenced polymer using a very periodic (or crystalline) template.
In summary, mineral catalysis must be rejected as a mechanism for doing either
the chemical or configurational entropy work required to polymerize the macromolecules
of life. It can only assist in polymerizing short, random chains of polymers
from selected high-energy biomonomers by assisting in doing the thermal entropy
work.
Nonlinear, Nonequilibrium Processes
1. Ilya Prigogine
Prigogine has developed a more general formulation of the
laws of thermodynamics which includes nonlinear, irreversible processes such as
autocatalytic activity. In his book Self
Organization in Nonequilibrium Systems (1977)19
co-authored with Nicolis, he summarized this work and its application to the
organization and maintenance of highly complex structures in living things. The
basic thesis in the book is that there are some systems which obey non-linear
laws---laws that produce two distinct kinds of behavior. In the neighborhood of
thermodynamic equilibrium, destruction of order prevails (entropy achieves a
maximum value consistent with the system constraints). If these same systems
are driven sufficiently far from equilibrium, however, ordering may appear
spontaneously.
Heat flow by convection is an example of this type of behavior. Heat conduction
in gases normally occurs by the random collision of gas molecules. Under
certain conditions, however, heat conduction may occur by a heat-convection
current---the coordinated movement of many gas molecules. In a similar way,
water flow out of a bathtub may occur by random movement of the water molecules
under the influence of gravity. Under certain conditions, however, this random
movement of water down the drain is replaced by the familiar soapy swirl---the
highly coordinated flow of the vortex. In each case random movements of
molecules in a fluid are spontaneously replaced by a highly ordered behavior.
Prigogine et al.,20 Eigen,21 and others have
suggested that a similar sort of self-organization may be intrinsic in organic
chemistry and can potentially account for the highly complex macromolecules
essential for living systems.
But such analogies have scant relevance to the origin-of-life question. A major
reason is that they fail to distinguish between order and complexity. The
highly ordered movement of energy through a system as in convection or vortices
suffers from the same shortcoming as the analogies to the static, periodic
order of crystals. Regularity or order cannot serve to store the large amount
of information required by living systems. A highly irregular, but specified,
structure is required rather than an ordered structure. This is a serious flaw
in the analogy offered. There is no apparent connection between the kind of spontaneous
ordering that occurs from energy flow through such systems and the work
required to build aperiodic information-intensive macromolecules like DNA and
protein. Prigogine, et al.22 suggest that the energy flow
through the system decreases the system entropy, leading potentially to the
highly organized structure of DNA and protein. Yet they offer no suggestion as
to how the decrease in thermal entropy from energy flow through the system
could be coupled to do the configurational entropy work required.
A second reason for skepticism about the relevance of the models developed by
Prigogine, et al.23 and others is that ordering produced
within the system arises through constraints imposed in an implicit way at the
system boundary. Thus, the system order, and more importantly the system
complexity, cannot exceed that of the environment.
Walton24 illustrates this concept in the following way. A container
of gas placed in contact with a heat source on one side and a heat sink on the
opposite side is an open system. The flow of energy through the system from the
heat source to the heat sink forms a concentration relative to the gas in the
cooler region. The order in this system is established by the structure:
source-intermediate systems-sink. If this structure is removed, allowing the
heat source to come into contact with the heat sink, the system decays back to
equilibrium. We should note that the information induced in an open system
doesn't exceed the amount of information built into the structural environment,
which is its source.
Condensation of nucleotides to give polynucleotides or nucleic acids can be
brought about with the appropriate apparatus (i.e., structure) and supplies of
energy and matter. Just as in Walton's illustration, however, Mora25has
shown that the amount of order (not to mention specified complexity) in the
final product is no greater than the amount of information introduced in the
physical structure of the experiment or chemical structure of the reactants.
Non-equilibrium thermodynamics does not account for this structure, but assumes
it and then shows the kind of organization which it produces. The origin and
maintenance of the structure are not explained, and as Harrison26
correctly notes this question leads back to the origin of structure in the
universe. Science offers us no satisfactory answer to this problem at present.
Nicolis and Prigogine27 offer their trimolecular model as an example
of a chemical system with the required nonlinearity to produce self ordering.
They are able to demonstrate mathematically that within a system that was
initially homogeneous, one may subsequently have a periodic, spatial variation
of concentration. To achieve this low degree of ordering, however, they must
require boundary conditions that could only be met at cell walls (i.e., at
membranes), relative reaction rates that are atypical of those observed in
condensation reactions, a rapid removal of reaction flow products, and a
trimolecular reaction (the highly unlikely simultaneous collision of three
atoms). Furthermore the trimolecular model requires chemical reactions that are
essentially irreversible. But condensation reactions for polypeptides or
polynucleotides are highly reversible unless all water is removed from the
system.
They speculate that the low degree of spatial ordering achieved in the simple
trimolecular model could potentially be orders of magnitude greater for the
more complex reactions one might observe leading up to a fully replicating
cell. The list of boundary constraints, relative reaction rates, etc. would,
however, also be orders of magnitude larger. As a matter of fact, one is left
with so constraining the system at the boundaries that ordering is inevitable
from the structuring of the environment by the chemist. The fortuitous
satisfaction of all of these boundary constraints simultaneously would be a its
miracle in its own right.
It is possible at present to synthesize a few proteins such as insulin in the
laboratory. The chemist supplies not only energy to do the chemical and thermal
entropy work, however, but also the necessary chemical manipulations to
accomplish the configurational entropy work. Without this, the selection of the
proper composition and the coding for the right sequence of amino acids would
not occur. The success of the experiment is fundamentally dependent on the
chemist.
Finally, Nicolis and Prigogine have postulated that a system of chemical
reactions which explicitly shows autocatalytic activity may ultimately be able
to circumvent the problems now associated with synthesis of prebiotic DNA and
protein. It remains to be demonstrated experimentally, however, that
these models have any real correspondence to prebiotic condensation reactions.
At best, these models predict higher yields without any mechanism to control
sequencing. Accordingly, no experimental evidence has been reported to show how
such models could have produced any significant degree of coding. No, the
models of Prigogine et al., based on non-equilibrium thermodynamics, do
not at present offer an explanation as to how the configurational entropy work
is accomplished under prebiotic conditions. The problem of how to couple energy
flow through the system to do the required configurational entropy work
remains.
2. Manfred Eigen
In his comprehensive application of nonequilibrium
thermodynamics to the evolution of biological systems, Eigen28 has
shown that selection could produce no evolutionary development in an open
system unless the system were maintained far from equilibrium. The reaction
must be autocatalytic but capable of self-replication. He develops an argument
to show that in order to produce a truly self-replicating system the
complementary base-pairing instruction potential of nucleic acids must be
combined with the catalytic coupling function of proteins. Kaplan29
has suggested a minimum of 20-40 functional proteins of 70-100 amino acids
each, and a similar number of nucleic acids would be required by such a system.
Yet as has previously been noted, the chance origin of even one protein of 100
amino acids is essentially zero.
The shortcoming of this model is the same as for those previously discussed;
namely, no way is presented to couple the energy flow through the system to
achieve the configurational entropy work required to create a system capable of
replicating itself.
Periodically we see reversions (perhaps inadvertent ones) to chance in the
theoretical models advanced to solve the problem. Eigen's model illustrates
this well. The model he sets forth must necessarily arise from chance events
and is nearly as incredible as the chance origin of life itself. The fact that
generally chance has to be invoked many times in the abiotic sequence has been
called by Brooks and Shaw "a major weakness in the whole chemical evolutionary
theory."30
Experimental Results in Synthesis of Protein and DNA
Thus far we have reviewed the various theoretical models
proposed to explain how energy flow through a system might accomplish the work
of synthesizing protein and DNA macromolecules, but found them wanting.
Nevertheless, it is conceivable that experimental Support for a spontaneous
origin of life can be found in advance of the theoretical explanation for how
this occurs. What then can be said of the experimental efforts to synthesize
protein and DNA macromolecules? Experimental efforts to this end have been
enthusiastically pursued for the past thirty years. In this section, we will
review efforts toward the prebiotic syntheses of both protein and DNA,
considering the three forms of energy flow most commonly thought to have been
available on the early earth. These are thermal energy (volcanoes), radiant
energy (sun), and chemical energy in the form of either condensing agents or
energy-rich precursors. (Electrical energy is excluded at this stage of
evolution as being too "violent," destroying rather than joining the
biomonomers.)
Thermal Synthesis
Sidney Fox31 has pioneered the thermal synthesis of polypeptides,
naming the products of his synthesis proteinoids. Beginning with either an
aqueous solution of amino acids or dry ones, he heats his material at 2000oC
for 6-7 hours.
[NOTE: Fox has modified this picture in recent years by
developing "low temperature" syntheses, i.e., 90-120oC.
See S. Fox, 1976. J Mol Evol
8, 301; and D. Rohlfing, 1976. Science
193, 68].
All initial solvent water, plus water produced during
Polymerization, is effectively eliminated through vaporization. This
elimination of the water makes possible a small but significant yield of
polypeptides, some with as many as 200 amino acid units. Heat is introduced
into the system by conduction and convection and leaves in the form of steam.
The reason for the success of the polypeptide formation is readily seen by
examining again equations 8-15 and 8-16. Note that increasing the temperature
would increase the product yield through increasing the value of exp (- G / RT. But more
importantly, eliminating the water makes the reaction irreversible, giving an
enormous increase in yield over that observed under equilibrium conditions by
the application of the law of mass action.
Thermal syntheses of polypeptides fail, however, for at least four reasons.
First, studies using nuclear magnetic resonance (NMR) have shown that thermal
proteinoids "have scarce resemblance to natural peptidic material because
beta, gamma, and epsilon peptide bonds largely predominate over alpha-peptide
bonds."32
[NOTE: This quotation refers to peptide links involving the
beta-carboxyl group of aspartic acid, the gamma-carboxyl group of glutamic
acid, and the epsilon-amino group of lysine which are never found in natural
proteins. Natural proteins use alpha-peptide bonds exclusively].
Second, thermal proteinoids are composed of approximately
equal numbers of L- and D-amino acids in contrast to viable proteins with all
L-amino acids. Third, there is no evidence that proteinoids differ
significantly from a random sequence of amino acids, with little or no
catalytic activity. [It is noted, however, that Fox has long disputed this.]
Miller and Orgel have made the following observation with regard to Fox's claim
that proteinoids resemble proteins:
The degree of nonrandomness in thermal polypeptides so far
demonstrated is minute compared to nonrandomness of proteins. It is deceptive,
then, to suggest that thermal polypeptides are similar to proteins in their
nonrandomness.33
Fourth, the geological conditions indicated are too
unreasonable to be taken seriously. As Folsome has commented, "The central
question [concerning Fox's proteinoids] is where did all those pure, dry,
concentrated, and optically active amino acids come from in the real,
abiological world?"34
There is no question that thermal energy flow through the system including the
removal of water is accomplishing the thermal entropy and chemical work
required to form a polypeptide (300 kcal/mole in our earlier example). The fact
that polypeptides are formed is evidence of the work done. It is equally clear
that the additional configurational entropy work required to convert an
aperiodic unspecified polypeptide into a specified, aperiodic polypeptide which
is a functional protein has not been done (159 kcal/mole in our earlier
example).
It should be remembered that this 159 kcal/mole of configurational entropy work
was calculated assuming the sequencing of the amino acids was the only
additional work to be done. Yet the experimental results of Temussi et al.,35
indicate that obtaining all Lamino acids from a racemic mixture and getting
alpha-linking between the amino acids are quite difficult. This requirement
further increases the configurational entropy work needed over that estimated
to do the coding work (159 kcal/mole). We may estimate the magnitude of this
increase in the configurational entropy work term by returning to our original
calculations (eq. 8-7 and 8-8).
In our original calculation for a hypothetical protein of 100 amino acid units,
we assumed the amino acids were equally divided among the twenty types. We calculated
the number of possible amino acid sequences as follows:
cr = 100! / 5!
5! 5!....5! = 100! / (5!)20 = 1.28 x 10115 (9-3)
If we note that at each site the probability of having an L-amino acid is
50%, and make the generous assumption that there is a 50% probability that a given
link will be of the alpha-type observed in true proteins, then the number of
ways the system can be arranged in a random chemical reaction is given by
cr = 1.28 x 10115
x 2100 x 299 = 10175 (9-4)
where 2100 refers to the number of additional arrangements
possible, given that each site could contain an L- or D-amino acid, and 299
assumes the 99 links between the 100 amino acids in general are equally divided
between the natural alpha-links and the unnatural beta-, gamma-, or
epsilon-links.
[NOTE Some studies indicate less than 50% alpha-links in
peptides formed by reacting random mixtures of amino acids. (P.A. Temussi, L.
Paolillo, F.E. Benedetti, and S. Andini, 1976. J. Mol. Evol. 7, 105.)].
The requirements for a biologically functional protein
molecule are: (1) all L-amino acids, (2) all alpha-links, and (3) a specified
sequence. This being so, the calculation of the configurational entropy of the
protein molecule using equation 8-8 is unchanged except that the number of ways
the system can be arranged, (cr), is
increased from 1.28 x 10115 to 1.0 x 10175 as shown in
equations 9-3 and 9-4. We may use the relationships of equations 8-7 and 8-8
but with the number of permutations modified as shown here to find a total
configurational entropy work. When we do, we get a total configurational
entropy work of 195 kcal/mole, of which 159 kcal/mole is for sequencing and 36
kcal/mole to attain all L-amino acids and all alpha-links. Finally, it should
be recognized that Fox and others who use his approach avoid a much larger
configurational entropy work term by beginning with only amino acids, i.e.,
excluding other organic chemicals and thereby eliminating the "selecting
work" which is not accounted for in the 195 kcal/mole calculated above.
In summary, undirected thermal energy is only able to do the chemical and
thermal entropy work in polypeptide synthesis, but not the coding (or
sequencing) portion of the configurational entropy work. Protenoids are just
globs of random polymers. That a polymer composed exclusively of amino acids
(but without exclusively peptide bonds) was formed is a result of the fact that
only amino acids were used in the experiment. Thus, the portion of the
configurational entropy work that was done---the selecting work---was
accomplished not by natural forces but by illegitimate investigator
interference. It is difficult to imagine how one could ever couple random
thermal energy flow through the system to do the required configurational
entropy work of selecting and sequencing. Finally, this approach is of very
questionable geological significance, given the many fortuitous events that are
required, as others have noted.
Solar Energy
Direct photochemical (UV) polymerization reactions to form polypeptides and
polynucleotides have occasionally been discussed in the literature. The idea is
to drive forward the otherwise thermodynamically unfavorable polymerization
reaction by allowing solar energy to flow through the aqueous system to do the
necessary work. It is worth noting that minor yields of small peptides can be
expected to form spontaneously, even though the reaction is unfavorable (see
eq. 8-16), but that greater yields of larger peptides can be expected only if
energy is somehow coupled to the reaction. Fox and Dose have examined the
peptide results of Bahadur and Ranganayaki36 and concluded that UV
irradiation did not couple with the reaction. They comment, "The authors
do not show that they have done more than accelerate an approach to an
unfavorable equilibrium. They may merely have reaffirmed the second law of
thermodynamics."37 Other attempts to form polymers directly
under the influence of UV light have not been encouraging because of this lack
of coupling. Neither the chemical nor the thermal entropy work, and definitely
not any configurational entropy work, has been accomplished using solar energy.
Chemical Energy (Energy-Rich Condensing Agents)
Through the use of condensing agents, the energetically unfavorable dipeptide
reaction (G1 = + 3000
cal/mole) is made energetically favorable (G3 < 0) by
coupling it with a second reaction which is sufficiently favorable
energetically (G2 < 0), to
offset the energy requirement of the dipeptide reaction:
dipeptide reaction
A - OH + H - B A - B + H20 G1 > 0 (9-5)
condensing agent reaction
C + H20 D G2 < 0 (9-6)
coupled reaction
A - OH + H - B + C A - B + D G3 < 0 (9-7)
As in thermal proteinoid formation, the free water is removed. However, in
this case, it is removed by chemical reaction with a suitable poly- condensing
agent-one which has a sufficient decrease in Gibbs free energy to drive the
reaction forward (i.e., G2 0 and | G2 | |G1 | so that G1 + G2 = G3 0.
Unfortunately, it has proved difficult to find condensing agents work. for
these macromolecule syntheses that could have originated on the primitive earth
and functioned properly under mild conditions in an aqueous environment.38
Meanwhile, other condensing agents which are not prebiotically significant (e.g.,
polymetaphosphates) are used in experiments. The plausible cyanide derivative
candidates for condensing agents on the early earth hydrolyze readily in
aqueous solutions (see Chapter 4). In the process, they do not couple
preferentially with the H20 from the condensation-dehydration
reaction. Condensing agents observed in living systems today are produced only
by living systems, and thus are not prebiotically significant. Moreover, enzyme
activity in living systems first activates amino acids and then brings about
condensation of these activated species, thus avoiding the problem of
indiscriminate reaction with water.
Notice that if we could solve the very significant problems associated with the
prebiotic synthesis of polypeptides by using condensing agents, we would still
succeed only in polymerizing random polypeptides. Only the chemical and thermal
entropy work would be accomplished by an appropriate coupling of the condensing
agent to the condensation reaction. There is no reason to believe that condensing
agents could have any effect on the selecting or sequencing of the amino acids.
Thus, condensing agents are eliminated as a possible means of doing the
configurational entropy work of coding a protein or DNA.
Chemical Energy (Energy-Rich Precursors)
Because the formation of even random polypeptides from amino acids is so
energetically unfavorable (G = 300 kcal/mole for 100
amino acids), some investigators have attempted to begin with energy-rich
precursors such as HCN and form polypeptides directly, a scheme which is
"downhill" energetically, i.e., G < 0. There are
advantages to such an approach; namely, there is no chemical work to be done
since the bonding energy actually decreases as the energy-rich precursors react
to form more complex molecules. This decrease in bonding energy will drive the
reaction forward, effectively doing the thermal entropy work as well. The fly
in the ointment, however, is that the configurational entropy work is enormous
in going from simple molecules (e.g., HCN) directly to complex polymers in a
single step (without forming intermediate biomonomers).
The stepwise scheme of experiments is to react gases such as methane, ammonia,
and carbon dioxide to form amino acids and other compounds and then to react
these to form polymers in a subsequent experiment. In these experiments the
very considerable selecting-work component of the configurational entropy work
is essentially done by the investigator who separates, purifies, and
concentrates the amino acids before attempting to polymerize them. Matthews39
and co-workers, however, have undertaken experiments where this intermediate
step is missing and the investigator has no opportunity to contribute even
obliquely to the success of the experiment by assisting in doing the selecting
part of the configurational entropy work. In such experiments-undoubtedly more
plausible as true prebiotic simulations-the probability of success is, however,
further reduced from the already small probabilities previously mentioned.
Using HCN as an energy-rich precursor, and ammonia as a catalyst, Matthews and
Moser40 have claimed direct synthesis of a large variety of
chemicals under anhydrous conditions. After treating the polymer with water,
even peptides are said to be among the products obtained. But as Ferris et
al.,41 have shown, the HCN polymer does not release amino acids
upon treatment with proteolytic (protein splitting) enzymes; nor does it give a
positive biuret reaction (color test for peptides). In short, it is very hard
to reconcile these results with a peptidic structure.
Ferris42 and Matthews43 have agreed that direct synthesis
of polypeptides has not yet been demonstrated. While some peptide bonds may
form directly, it would be quite surprising to find them in significant
numbers. Since HCN gives rise to other organic compounds, and various kinds of
links are possible, the formation of polypeptides with exclusively alpha-links
is most unlikely. Furthermore, no sequencing would be expected from this
reaction, which is driven forward and "guided" only by chemical
energy.
While we do not believe Matthews or others will be successful in demonstrating
a single step synthesis of polypeptides from HCN, this approach does involve
the least investigator interference, and thus, represents a very plausible
prebiotic simulation experiment. The approach of Fox and others, which involves
reacting gases to form many organic compounds, separating out amino acids,
purifying, and finally polymerizing them, is more successful because it
involves a greater measure of investigator interference. The selecting portion
of the configurational entropy work is being supplied by the scientist.
Matthew's lack of demonstrable success in producing polypeptides is a
predictable indication of the enormity of the problem of prebiotic synthesis
when it is not overcome by illegitimate investigator interference.
Mineral Catalysis
A novel synthesis of polypeptides has been reported44 which employs
mineral catalysis. An aqueous solution of energy-rich aminoacyl adenylates
(rather than amino acids) is used in the presence of certain layered clays such
as those known as montmorillonites. Large amounts of the energy-rich reactants
are adsorbed both on the surface and between the layers of clay. The catalytic
effect of the clay may result primarily from the removal of reactants from the
solution by adsorption between the layers of clay. This technique has resulted
in polypeptides of up to 50 units or more. Although polymerization definitely
occurs in these reactions, the energy-rich aminoacyl adenylate (fig. 9-1) is of
very doubtful prebiotic significance per the discussion of competing reactions
in Chapter 4. Furthermore, the use of clay with free amino acids will not give
a successful synthesis of polypeptides. The energy-rich aminoacyl adenylates
lower their chemical or bonding energy as they polymerize, driving the reaction
forward, and effectively doing the thermal entropy work as well. The role of
the clay is to concentrate the reactants and possibly to catalyze the
reactions. Once again, we are left with no apparent means to couple the energy
flow, in this case in the form of prebiotically questionable energy-rich
precursors, to the configurational entropy work of selecting and sequencing
required in the formation of specified aperiodic polypeptides, or proteins.
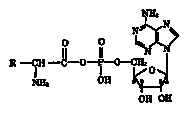
Figure 9-1.
Aminoacyl adenylate.
Summary of Experimental Results on Prebiotic Synthesis of protein
In summary, we have seen that it is possible to do the thermal entropy work and
chemical work necessary to form random polypeptides, e.g., Fox's proteinoids.
In no case, though, has anyone been successful in doing the additional
configurational entropy work of coding necessary to convert random polypeptides
into proteins. Virtually no mechanism with any promise for coupling the random
flow of energy through the system to do this very specific work has come to
light. The prebiotic plausibility of the successful synthesis of polypeptides
must be questioned because of the considerable configurational entropy work of
selecting done by the investigator prior to the polymer synthesis. Surely no
suggestion is forthcoming that the right composition of just the subset of
amino acids found in living things was "selected" by natural means,
or that this subset consists only of L-a-amino acids. This is precisely why a
large measure of the credit in forming proteinoids must go to Fox and others
rather than nature.
Summary of Experimental Results on Prebiotic Synthesis of DNA
The prebiotic synthesis of DNA has proved to be even more difficult than that
of protein. The problems that beset protein synthesis apply with greater force
to DNA synthesis. Energy flow through the system may cause the nucleotides to
chemically react and form a polymer chain, but it is very difficult to get them
to attach themselves together in a specified way. For example, 3' - 5' links on
the sugar are necessary for the DNA to form a helical structure (see fig. 9-2).
Yet 2'-5' links predominate in most prebiotic simulation experiments.45
The sequencing of the bases in DNA is also crucial, as is the amino acid
sequence in proteins. Both of these requirements are problems in doing the
configurational entropy work. It is one thing to get molecules to chemically
react; it is quite another to get them to link up in the right arrangement. To
date, researchers have only succeeded in making oligonucleotides, or relatively
short chains of nucleotides, with neither consistent 3'-5' links nor specific
base sequencing.
Figure 9-2.
A section from a DNA chain showing the sequence AGCT.
Miller and Orgel summarized their chapter on prebiotic condensation
reactions by saying:
This chapter has probably been confusing to the reader. We
believe that is because of the limited progress that has been made in the study
of prebiotic condensation. Many interesting scraps of information are
available, but no correct pathways have yet been discovered.46
The situation is much the same today.
Summary Discussion of Experimental Results
There is an impressive contrast between the considerable success in
synthesizing amino acids and the consistent failure to synthesize protein and
DNA. We believe the reason is the large difference in the magnitude of the
configurational entropy work required. Amino acids are quite simple compared to
protein, and one might reasonably expect to get some yield of amino acids, even
where the chemical reactions that occur do so in a rather random fashion. The
same approach will obviously be far less successful in reproducing complex
protein and DNA molecules where the configurational entropy work term is a
nontrivial portion of the whole. Coupling the energy flow through the system to
do the chemical and thermal entropy work is much easier than doing the
configurational entropy work. The uniform failure in literally thousands of
experimental attempts to synthesize protein or DNA under even questionable
prebiotic conditions is a monument to the difficulty in achieving a high degree
of information content, or specified complexity from the undirected flow
of energy through a system.
We must not forget that the total work to create a living system goes far
beyond the work to create DNA and protein discussed in this chapter. As we
stated before, a minimum of 20-40 proteins as well as DNA and RNA are required
to make even a simple replicating system. The lack of known energy-coupling
means to do the configurational entropy work required to make DNA and protein
is many times more crucial in making a living system. As a result, appeals to
chance for this most difficult problem still appear in the literature in spite
of the fact that calculations give staggeringly low probabilities, even on the
scale of 5 billion years. Either the work---especially the organizational
work---was coupled to the flow of energy in some way not yet understood, or
else it truly was a miracle.
Summary of Thermodynamics Discussion
Throughout Chapters 7-9 we have
analyzed the problems of complexity and the origin of life from a thermodynamic
point of view. Our reason for doing this is the common notion in the scientific
literature today on the origin of life that an open system with energy and mass
flow is a priori a sufficient explanation for the complexity of life. We
have examined the validity of such an open and constrained system. We found it
to be a reasonable explanation for doing the chemical and thermal entropy work,
but clearly inadequate to account for the configurational entropy work of
coding (not to mention the sorting and selecting work). We have noted the need
for some sort of coupling mechanism. Without it, there is no way to convert the
negative entropy associated with energy flow into negative entropy associated
with configurational entropy and the corresponding information. Is it
reasonable to believe such a "hidden" coupling mechanism will be
found in the future that can play this crucial role of a template, metabolic
motor, etc., directing the flow of energy in such a way as to create new
information?
References
1. Albert L. Lehninger, 1970. Biochemistry.
2. H.P. Yockey, 1977. J. Theoret. Biol.
67, 377; R.W. Kaplan, 1974. Rad. Environ. Biophys. 10, 31.
3. M. Eigen, 1971. Die Naturwiss. 58,
465.
4. G. Steinman, 1967. Arch. Biochem.
Biophys. 121, 533. 5. A.G. Cairns-Smith, 1971. The Life Puzzle.
6. F. Crick, 1966. Of Molecules and Men.
7. Eigen, Die Naturwiss., p.
465; S.L. Miller and L.E. Orgel, 1974. The
Origins of Life on the Earth.
8. J.B.S. Haldane, 1965. In The Origins
of Prebiological Systems and of Their Molecular Matrices, ed. S.W.
Fox.
9. T. Dobzhansky, 1965. In The Origins of
Prebiological Systems and of Their Molecular Matrices, p.310.
10. Ludwig von Bertalanffy, 1967. Robots,
Men and Minds.
11. G. Steinman and M. Cole, 1967. Proc.
Nat. Acad. Sci. U.S. 58, 735; Steinman, Arch. Biochem. Biophys. , p.533.
12. A. Katchalsky, 1973. Die Naturwiss.
60,215; M. Calvin, 1975. Amer. Sci.
63, 169; C.E. Folsome, 1979. The
Origin of Life.
13. Steinman, Arch. Biochem. Biophys. 121,
533; Steinman and Cole, Proc. Nat. Acad.
Sci. U.S. 5, p.735.
14. H.P. Yockey, 1981. J. Theoret. Biol
91, 13.
15. Katchalsky, Die Naturwiss., p.215.
16. H.R. Hulett, 1969. J. Theoret. Biol
24, 56.
17. Katchalsky, Die Naturwisa., p.216.
18. A.E. Wilder-Smith, 1970. The Creation
of Life.
19. G. Nicolis and
20.
21. Eigen, Die Naturwiss., p.465.
22. Prigogine, Nicolis, and Babloyantz, Physics
Today, p.23-31.
23. Ibid; Nicolis and Prigogine, Self Organization
in Nonequilibrium Systems.
24. J.C. Walton, 1977. Origins, 4,
16.
25. P.T. Mora, 1965. In The Origins of
Prebiological Systems and of Their Molecular Matrices, p.39.
26. E.R. Harrison, 1969. In Hierarchical
Structures. ed. L.L. Whyte, A.G. Wilson, and D. Wilson, New York:
Elsevier, p.87.
27. Nicolis and Prigogine, Self
Organization in Nonequilibrium Systems.
28. Eigen, Die Naturwiss., p.465; 1971. Quart.
Rev. Biophys. 4, 149.
29. Kaplan, Rad. Environ. Biophysics, p.31.
30. J. Brooks and G. Shaw, 1973. Origin
and Development of Living Systems.
31. S.W. Fox and K. Dose, 1977. Molecular
Evolution and the Origin of Life.
32. P.A. Temussi, L. Paolillo, L. Ferrera, L. Benedetti, and S. Andini, 1976. J. Mol Evol 7, 105.
33. S.L. Miller and L.E. Orgel, 1974. The
Origins of Life on Earth
34. C.E. Folsome, 1979. The Origin of
Life.
35. Temussi, Paolillo, Ferrera, Benedetti, and Andini, J. Mol. Evol., p.105.
36. K. Bahadur and S. Ranganayaki, 1958. Proc.
Nat. Acad. Sci. (
37. S.W. Fox and K. Dose, 1972. Molecular
Evolution and the Origin of Life.
38. J. Hulshof and C. Ponnamperuma, 1976. Origins
of Life 7, 197.
39. C.N. Matthews and R.E. Moser, 1966. Proc.
Nat. Acad. Sci. U.S. 56, 1087; C.N. Matthews, 1975. Origins of Life 6, 155; C.
Matthews, J. Nelson, P. Varma, and R. Minard, 1977. Science 198 622; C.N. Matthews,
1982. Origins of Life 12,
281.
40. C.N. Matthews, and R.E. Moser, 1967. Nature
215,1230.
41. J.P. Ferris, D.B. Donner, and A.P. Lobo, 1973. J. Mol Biol. 74, 499.
42. J.P. Ferris, 1979. Science 203,
1135.
43. C.N. Matthews, 1979. Science
203, 1136.
44. Katchalsky, Die Naturwiss., p.215.
45. R.E. Dickerson, September 1978. Sci.
Amer., p.70.
46. Miller and Orgel, The Origins of Life
on the Earth, p.148.